本日2月23日を3ケタの数で表した223は素数です。西暦を含めた20190223は、2161×9343と素因数分解できるので、素数ではありません。
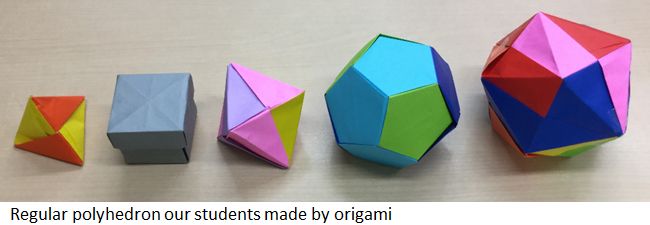
多角形で囲まれた立体を多面体と言います。その中で、1種類の正多角形(正三角形、正方形、正五角形・・・)の面で作られたものをとくに「正多面体」と呼んでいます。正多面体はたった5種類しかありません。正四面体、正六面体(立方体)、正八面体、正十二面体、正二十面体です。そのうち、正四面体、正八面体、正二十面体は、正三角形の面でできています。立方体(正六面体)は正方形で、正十二面体は正五角形でできています。写真は、左から、正四面体、正六面体(立方体)、正八面体、正十二面体、正二十面体です。2階数学メディアスペースに模型を展示しています。

正六面体は普通のサイコロ、ルービックキューブなどよく見かけると思います。正八面体は映画「天空の城ラピュタ」で、ラピュタの中心部にある巨大な「飛行石」の形と言えば思い浮かぶ方が多いのではないでしょうか。
これらの正多面体は、かなり昔から知られていたと言われています。プラトン(紀元前427-紀元前347)が彼の著書「ティマイオス」で正多面体について触れています。それで、正多面体は「プラトンの立体」とも名付けられています。
正多面体が5種類しかないことは、それぞれの正多面体の角が正〇角形何枚でできているかを考えてみるとわかってきます。
①正四面体 - 正三角形が3枚
②正六面体 - 正方形が3枚
③正八面体 - 正三角形が4枚
④正十二面体 - 正五角形が3枚
⑤正二十面体 - 正三角形が5枚
ヒントは2つです。1つ目は、正多角形が3枚以上でないと立体の角を作ることはできません。もう1つは、角に集まる正多角形の角度の合計は360度未満でなければなりません。ここから先は、ぜひ考えてみてください。
(数学科 園田)
“Only 5 kinds of Regular polyhedron!”
Polyhedron are solids with flat polygonal faces. There are special polyhedron called ‘Regular polyhedron’ which are made with the same regular polygons with the same number of faces meeting at each vertex. Only 5 kinds of solids meet these criteria. Tetrahedron, cube, octahedron, dodecahedron and icosahedron. Those are found on the second floor in our math space.
You will often see a cube as a dice and a Rubik’s cube. I think you saw a octahedron as a big Levistone in the Japanese movie ‘Castle in the Sky’.
People in old times knew about regular polyhedron. Plato described about them in his book ‘Timaeus’. So we also call regular polyhedron a Platonic solid.
We can explain that there are only 5 kinds of regular polyhedron by thinking how the structure of the vertices of regular polyhedron are made.
(1)Tetrahedron – 3 regular triangles
(2)Cube – 3 squares
(3)Octahedron – 4 regular triangles
(4)Dodecahedron – 3 regular pentagons
(5)icosahedron – 5 triangles
We have two hints. One is that we can make veritices by more than 3 polygons. The other is that the sum of angles of regular polygons gathering at a point are less than 360 degrees.
Let’s think beyond here!
by Tsuyoshi Sonoda (Math Dept.)