今日9月7日を3ケタの数で表すと、907は素数です。西暦を含めた20190907は11×11×166867と素因数分解できるので、素数ではありません。
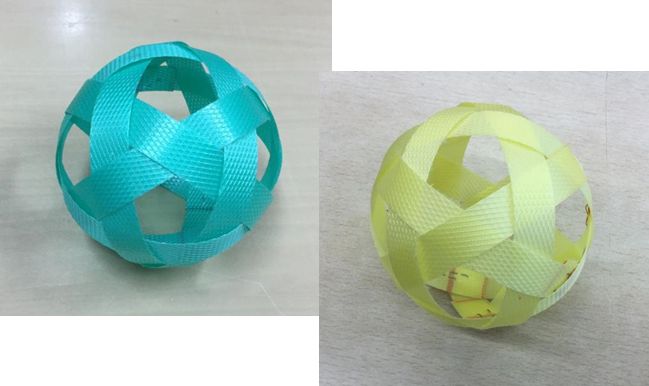
前回、工作用紙で自作したサッカーボール（「角切り（かくぎり）二十面体」という「半正多面体」の1つ）をご紹介しましたが、小包の包装などに使われているppバンドからも作ることができます。
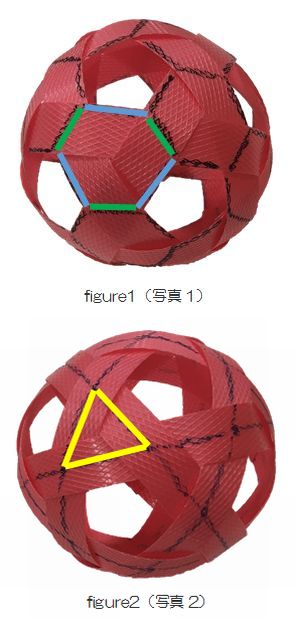
写真1を見てください。球体の表面に空いているすき間は正五角形です。そして、ppバンドを見ると、正五角形の各辺（青い線）とその頂点どうしを結ぶ線（緑の線）が正六角形を作っているのがおわかりになるでしょう。
ppバンドで作ったこの立体の不思議なところは、もう1種類の半正多面体に見えるところです。
写真2を見てください。ｐｐバンドの真ん中にマジックで線を引いていきます。こうすると、この立体は正五角形と正三角形でできた半正多面体に見えることがわかりますね。これは「二十・十二面体」と呼んでいる半正多面体です。
角切り二十面体（写真1）と二十・十二面体（写真2）、この2つの半正多面体は少し違いがあります。角切り二十面体の辺は、正五角形と正六角形にはさまれた辺（青い線）と2つの正六角形にはさまれた辺（緑の辺）、2通りの場合があります。一方、二十・十二面体は、すべての辺が正五角形と正三角形にはさまれています。それで、二十・十二面体は、半正多面体の中でも特別に「準正多面体」と呼ばれて区別されています。
（数学科 園田毅）
“Let’s make a soccer ball using PP bands 1”
I introduced a solid of a soccer ball made by working paper and it is called a “Truncated icosahedron” which is one type of ”semi – regular polyhedron” in the last blog. Today, I will show that we can also make the solid using PP bands. Please look at figure 1. The shape of holes on the surface of the sphere is a regular pentagon. If you check the PP bands, you will know that each side (blue lines) of regular pentagons and each other side (green lines) connecting their vertex make a regular hexagon.
A strange and interested thing about this solid using PP bands is that we can look it as other type of ”semi – regular polyhedron”.
Please look at figure 2. We write lines on the central part of the PP bands. Then you get that this solid is also a ”semi – regular polyhedron” made of regular pentagon and regular triangles. We call it a “Icosidodecahedron”.
There is a little defference between truncated icosahedron (figure 1) and icosidodecahedron (figure 2).
A truncated icosahedron has 2 types of the sides. One type (blue lines) are sandwiched between a regular pentagon and regular hexagon and another type (green lines) are sandwiched 2 regular hexagon. On the other hand, all sides (yellow lines) of icosidodecahedron are sandwiched between a regular pentagon and regular triangle. Then icosidodecahedron are distinguished from other semi – regular polyhedron and called “quasi – regular polyhedron”.
by Tsuyoshi Sonoda (Math Dept.)
