今日4月21日を3ケタの数で表すと、421は素数です。西暦を含めた20190421も素数になります。
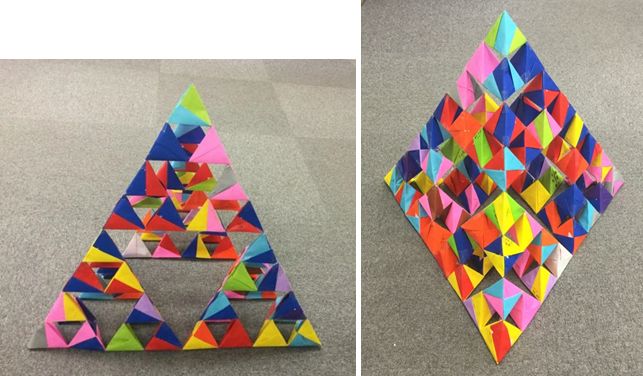
今回作ったシェルピンスキー四面体の体積は元の正四面体4つ分で、シェルピンスキー四面体と同じ大きさの正四面体の体積は元の正四面体8つ分なので、体積比は1：2であることを紹介しました。
シェルピンスキー四面体は正四面体の内部に穴が開いた立体なのですが、前回のブログで、内部の形がどうなっているか皆さんに質問をしました。実は、同じ折り紙ユニットで作った正八面体がぴったり入るのです。つまり、正八面体の体積は同じ辺の長さの正四面体4つ分であることがわかります。
私たちは、小さな正四面体を合体してシェルピンスキー四面体を作りましたが、実際のシェルピンスキー四面体は正四面体の内側に連続して穴を開けてできるイメージです。左の写真は本校の近くにある京都大学総合博物館に展示されているシェルピンスキー四面体の模型です。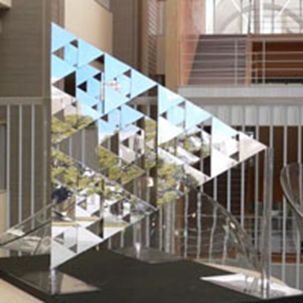
また、京都大学「シェルピンスキーの森」（写真下）をはじめ、日本各地にこの図形を利用した日よけが設置されています。（ららぽーと豊洲、東名阪自動車道御在所サービスエリアなど）太陽の光がこの図形を通ると、木漏れ日のある光景が現れ、暑さが和らぐそうです。「フラクタル日よけ」と呼んでいます。海外では2019年1月にオープンした台湾・台南市美術館2号館（日本の建築家 坂茂氏が設計）に、フラクタル日よけの屋根が採用されているそうです。
シェルピンスキー四面体は、とても不思議でおもしろい図形ですね。ぜひ数学博物館の展示もご覧になってください。
（数学科 園田）
“Sierpinski tetrahedron 2”
In the last blog, we said that the volume of a Sierpinski tetrahedron is 4 times of an original regular tetrahedron and a ’big regular tetrahedron’ is 8 times of an original regular tetrahedron, then the ratio is 1:2.
I also had a question for you in the last blog. What form is the space in a Sierpinski tetrahedron ? The answer is a regular octahedron by the same origami units fits perfectly. We can get that a regular octahedron is 4 times as a small regular tetrahedron.
This time, we united with small regular tetrahedron and made a big Sierpinski tetrahedron. In fact, please imagine that you make holes in a regular tetrahedron continuously. This is a model of Sierpinski tetrahedron exhibited in Kyoto University in the right photo.
There are some sunshades using this figure in Japan, for example, ‘The forest of Sierpinski’ in Kyoto University near our school, the shopping area ‘LaLaport TOYOSU’ in Tokyo and Gozaisho Service Area on Higashimeihan Expressway in Mie prefecture, etc. After the light of sun goes through this sunshade, we can see the scene with filtered sunlight and the heat getting let up. It is called a ‘fractal sunshade’. Tainan Art Museum opened in January 2019, building No.2 adopted the roof of fractal sunshade overseas.
Sierpinski tetrahedron is a mysterious and interesting shape. Please check the exhibit in our Museum!
by Tsuyoshi Sonoda (Math Dept.)
